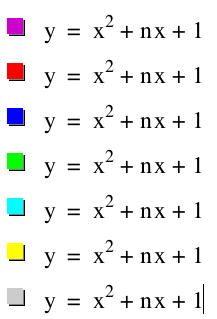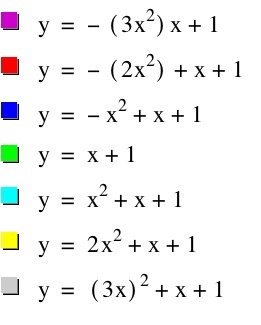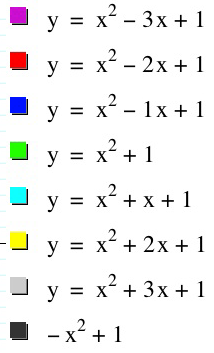Investigations of Graphs

by

Becky Mohl

 

Investigations of y = ax^2 + bx + c:

 

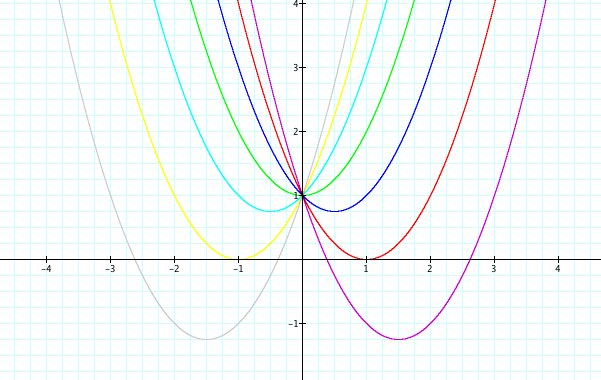
As we see in the above image, all the parabolas intersect at one point on the y-axis when b changes from -3 to 3.

However, what happens when we substitute n for b and see what happens when n changes from -infinity to infinity.

Here we see that when we let n go between -100 and 100 then the parabola still goes through the point (0,1) for all values of n. In the graph below, we see that the graph when n=-100 still goes through (0,1) when we zoom in and look at this point of intersection.

 

 

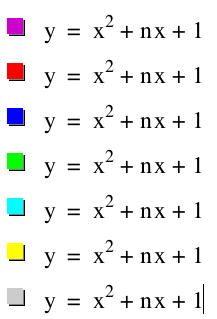
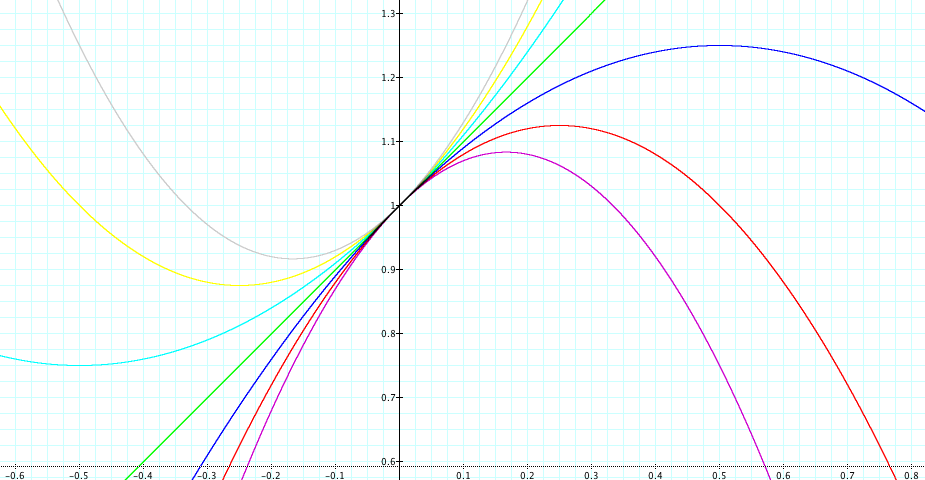
The same holds true for when n=100. We can see by the graph below.

 

So, we can make the assumption that anytime that a=1, c=1, and n=b from -100 to 100, the graph will always intersect at the point (0,1). This also implies that for any value of n, this will be the intersection as long as a and c remain constant.

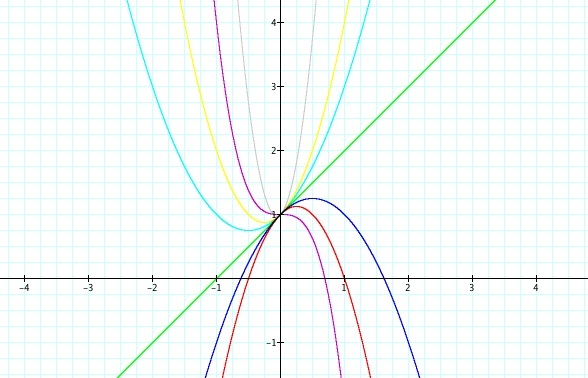
Now what happens if we keep c=1, b=1, and look what happens when a changes.

 

 

Still even with another variable changing, the graph still intersects at the point (o,1). Finally, lets look at what happens when a=1, b=1, and c changes.

 

 

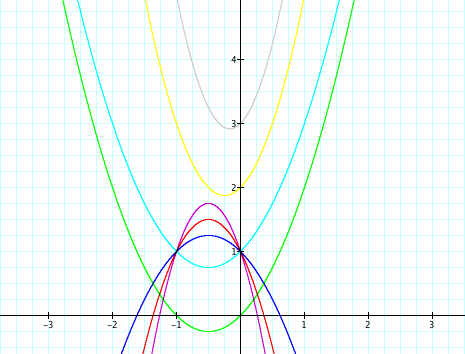
Now, finally we see that not all parabolas on the graph go through the point (0,1). Why is this? I believe that the graph when c changes is different because it is the only value without an x term in it. Because of this the graph is not squeezed as it normally does when multiplied by a constant. instead several of the parabolas are shifted up by the rules that shift graphs up, down, left, and right. This is very interesting to explore farther, and can lead to many extravagant figures.

Other interseting graphs ionclude:

 

....and

 

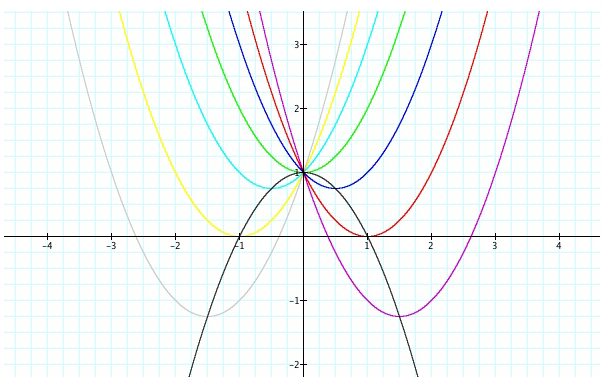
The bove graph was found by completing the sqyare: y= x^2 +bx + , and then finding the vertex of each parabola by the formula, (-b/2a, F(-b/2a).

Nect we find that our locus can be found at y = 1 - x^2 by using x = -b/2a since we know that his will be the maximum or minimum point depending on the direction of the curve, which in this case is negative so it is pontin downwards.

1. Complete square

y=(x+(b/2))^2 + ( 1- (b/2))^2.

2, Find vertex

3. Find the locus.

 

 

Back